9 The value of lim n → ∞ ( 1 n 1 1 n 2 1 6 n) is WBJEE 08 10 Here, x denotes the greatest integer less than or equal to x Given that f ( x) = x x The value obtained when this function is integrated with respect to x with lower limit as $$\lim_{n \rightarrow \infty} \dfrac{(sin(\dfrac{1}{n}))^2}{n^2})$$ Steps I have taken Getting rid of the square through the limit of a product is the product of it's limit so I will square the limit at the endThus ∑ n n 2 1 diverges by the Limit Comparison Test • If x =1 the series becomes ∞ ∑ n =1 (1) n1 n n 2 1 which converges by the Alternating Series Test since {n n 2 1} is a positive decreasing sequence with lim n →∞ n n 2 1 = 0 Thus, IOC = 1, 1) Observe While f (x) and f 0 (x) had the same radii of convergence

Limit Replace 1 By 0 Mathematics Stack Exchange
Lim (1 1/n^2)(1 2/n^2)...(1 n/n^2)
Lim (1 1/n^2)(1 2/n^2)...(1 n/n^2)-Lim 3−2n4n2 4n25n−3 lim 3 − 2 n 4 n 2 4 n 2 5 n − 3 có giá trị là bao nhiêu?Nếu limun = L lim u n = L thì lim 1 3√un8 lim 1 u n 8 3 có giá trị là bao
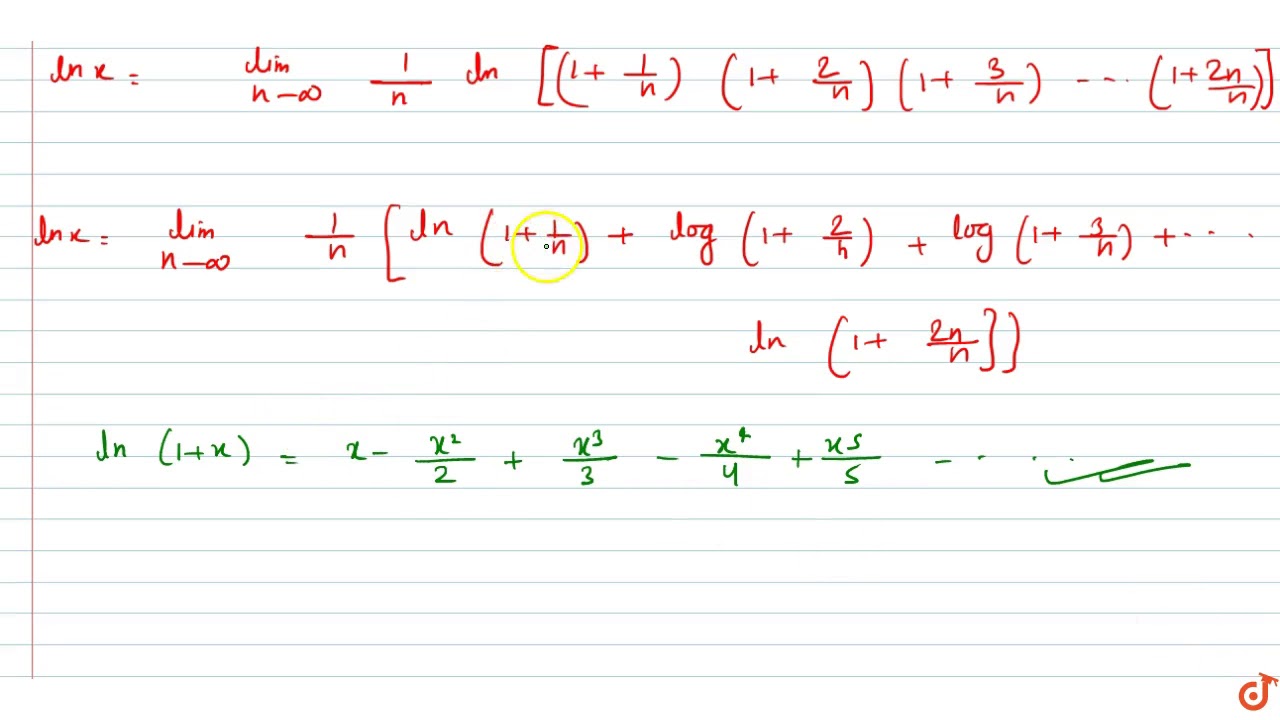



Lim N Gtoo N 1 N 2 3n N 2n 1 N Is Equal To Youtube
So in this case, the limit has to be zero, because the denominator approaches infinity WAY faster *I also just noticed that factoring out the 2 n will also take out every single term in the numerator 2n4 = 2 (n2), so goodbye n2 term;Lim N → ∞ 1 2 2 2 3 2 N 2 N 3 CBSE CBSE (Commerce) Class 11 Textbook Solutions 79 Important Solutions 14 Question Bank Solutions 6793 Concept Notes & Videos 3 Syllabus Advertisement Remove all ads Lim N → ∞ 1 2 2 2 3 2 N 2 N 3Stack Exchange network consists of 177 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange
Nếu limun = L lim u n = L thì lim√un 9 lim u n 9 có giá trị là bao nhiêu?\left( n 1 \right)!}{\left( n 2 \right)!If a_n = (1)^n(n^2)/(2n^2 2n 1), then lim a_n = (A) 1/2 (B) 1/2 0 (D) does not exist (E) None of these Previous question Next question Get more help from Chegg
Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreHomework Equations The Attempt at a Solution I believe it does converge because the higher power is in the denominator, so thus, it's limit is 0 Any help or hints on if I'm headed in the right direction would be very much appreciated!Lim n!1 n2 n2 1 = 1 Therefore, the term inside the arctangent is going to 1, so lim n!1 arctan n2 n2 1 = arctan(1) = ˇ 4 12Does the series X1 n=2 1 n2 p n converge or diverge?



Lim N 1 N 2 Sec 21 N2 2 N 2 Sec 24 N 2 1 Nsec 21 Equals Sarthaks Econnect Largest Online Education Community
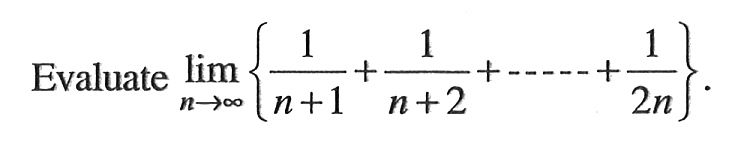



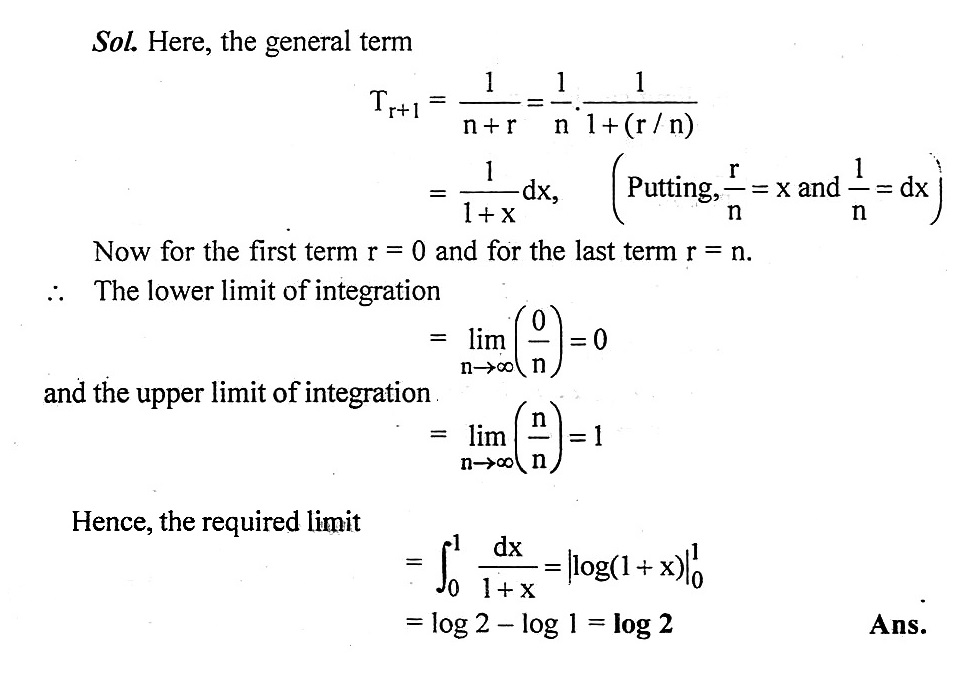
Evaluate Lim N 1 N 1 1 N 2 1 2n Mathematics 1 Question Answer Collection
Thank you in advanceShort Solution Steps \ { \frac { ( n 1 ) ( n 2 ) } { 2 n ^ { 2 } } \} { 2 n 2 ( n 1) ( n 2) } Use the distributive property to multiply n1 by n2 and combine like terms Use the distributive property to multiply n 1 by n 2 and combine like terms \frac {n^ {2}3n2} {2n^ {2}} 2 n 2 n 2 3 n 2`Lim_(n>oo)1/n^2 * sec^2 (1/n^2)2/n^2 * sec^2 (4/n^2)1/n * sec^2 1` JEE Main 21 4th session starts from Aug 26, application last date extended
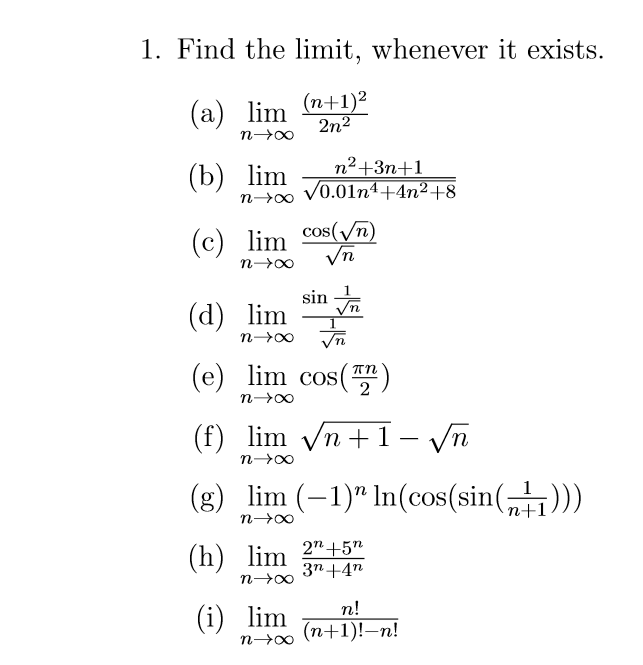



Find The Limit Whenever It Exists A Lim N Chegg Com




Sol 2 Functions And Mappings Complex Analysis
Value of lim n → ∞ (1 n 3 n 2 n 2 c o s n Elaborating n ln (1 n 3 n 2 n 2 c o s n ) = n ln (1 n 1 / n 2 c o s (n) / n 2Lim (1n22n23n2nn2) bằng A B 0 C 13 D 12Lim n ∞ ( 1 n 1 1 n 2 1 n 3 ⋯ 1 n 2 n ) Show transcribed image text Expert Answer Who are the experts?



Evaluate Lim N 1 N 1 1 N 2 1 2n Mathematics 1 Question Answer Collection




Tinh Giới Hạn Lim 2n 1 N 2 Lim 3n 2 1 N 2 4 Toan Học Lớp 11 Bai Tập Toan Học Lớp 11 Giải Bai Tập Toan
2n6 = 2 (n3), so goodbye n3 term, and it should go that way all the way downIn general, math\displaystyle\lim_{n\to\infty}\left(1\frac{x}{n}\right)^n=e^x/math Plugging in mathx=2/math math\displaystyle\lim_{n\to\infty}\left(1JEE Main 21 admit card for session 3 released Check important details related to the JEE Main 21 exam such as exam timing, venue, time slot etc




The Limit Does Not Exist Or Does It Ppt Download



Link Springer Com Content Pdf 10 1007 2f978 3 7091 6146 3 12 Pdf
\left( n 1 \right)!} \right\ \ = \lim_{n \to \infty Is the sequence {n/(n^21)} convergent, and if so, what is it's limit? Homework Statement find lim(n\\rightarrow\\infty (1/n^2 2/n^2 3/n^2 n1/n^2 ) Homework Equations b3 The Attempt at a Solution /b I could guess that the limit is zero but i dont know howto prove it



Www Jstor Org Stable




Show That Lim N Tends To Infinity 1 N 1 N 1 1 N 2 1 3n Log 3 Brainly In



0 件のコメント:
コメントを投稿